Some Considerations Concerning the Teaching of Negative Numbers
DOI:
10.37084/REMATEC.1980-3141.2023.n43.pe2023024.id502Palavras-chave:
Teaching of arithmetic, Cognitive obstacles, Negative numbers, Rule of signs, Inverse of the inverseResumo
The teaching of negative numbers is dealt with herein from the point of view of expanding the number classes from those containing only positive numbers to those that also include the negatives (and zero). The expansion involves generalizing the concept of “number” and making modifications in the definitions of the arithmetical operations. Each of these actions generates cognitive obstacles, both historically and pedagogically. In contrast to the historical situation, the concept of negative number is not problematic for the contemporary student because he/she encounters them with certain frequency in his/her daily life. The addition of negative numbers, especially in the case in which it becomes equivalent to subtraction in the natural numbers with the subtrahend greater than the minuend, is subject to a didactical obstacle, which can be overcome by using the number line as a visual/conceptual support. Also, the multiplication of two negative numbers, resulting in a positive number, constitutes a genuine epistemological obstacle due to the fact that the usual metaphors used to explain the negative numbers do not clarify this operation. The article thus proposes to consider double negatives as inverses of inverses and delineates a sequence of activities based on this metaphor to overcome the mentioned epistemological obstacle.
Downloads
Métricas
Referências
BACHELARD, Gaston. La formation de l’esprit scientifique. Paris: J. Vrin, 1938.
BARLOW, Peter. A New Mathematical and Philosophical Dictionary. London: G. and S Robinson, 1814.
EUCLID. The Thirteen Books of the Elements. Trans. Thomas L. Heath. 3 vols. New York: Dover, 1956.
FOSSA, John A. Os Primórdios da Teoria dos Números. 2 v. Natal: Editora da UFRN, 2010.
FOSSA, John A.; ANJOS, Marta Figueredo dos. Sobre a incompatibilidade dos números negativos com o conceito grego de ảrithmós. Revista Brasileira de História da Matemática, Rio Claro, SP, v. 7, n. 14, p. 163-171, 2007.
KUHN, Thomas. The Structure of Scientific Revolutions. Chicago: University of Chicago Press, 1962.
LUMPKIN, Beatrice. Zero in ancient Egypt? 2005. Disponível em: http://www. ethnomath.org/resources/ISGEm/084.htm. Acesso em 23/06/05.
MENDES, Iran Abreu; FOSSA, John A.; VALDÉS, Juan E. Nápoles. A História como um agente de cognição na Educação Matemática. Porto Alegre: Sulina, 2006.
PEACOCK, George. Treatise on Algebra. 2 vols. Mineola (NY): Dover, 2004.
PYCIOR, Helena M. Symbols, Impossible Numbers and Geometric Entanglements. Cambridge: University of Cambridge Press, 1997. DOI: https://doi.org/10.1017/CBO9780511895470
SCHUBRING, Gert. Números negativos: exemplos de obstáculos epistemológicos? São Paulo: Livraria da Física, 2018.
Downloads
Publicado
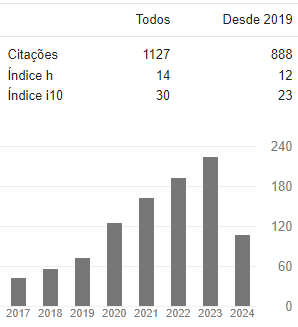
- Visualizações do Artigo 220
- PDF downloads: 189






 Português (Brasil)
Português (Brasil)
 Español (España)
Español (España)
 English
English