Estrategias asociadas al uso de GeoGebra en un contexto de resolución de problemas
DOI:
10.37084/REMATEC.1980-3141.2021.n37.p61-79.id252Palabras clave:
Resolución de Problemas, Geometría, GeoGebra, ArrastreResumen
RESUMEN
Este artículo presenta una experiencia en aula de matemática cuyo objetivo fue analizar cómo el uso constante de un Sistema de Geometría Dinámica (SGD), GeoGebra, en un contexto de resolución de problemas, permitió a un grupo de educadores matemáticos en formación utilizar y desarrollar estrategias de solución asociadas con el arrastre de objetos, medición de sus atributos y rastro de un punto como un medio para fomentar y desarrollar su razonamiento matemático. Los resultados indican que GeoGebra se convirtió en una herramienta que promovió nuevas formas de razonar a partir de la exploración dinámica. Las estrategias de solución permitieron a los participantes la visualización de patrones, la formulación y validación de conjeturas transitando desde el uso de argumentos visuales o empíricos hasta la construcción de un modelo algebraico o geométrico conectando conceptos de probabilidad, geometría euclidiana y de trigonometría.
Descargas
Métricas
Citas
GEERAERTS, L.; VENANT, F.; TANGUAY, D. Subterranean structures of technological tools and teaching issues in geometry. In: Proceedings of EDULEARN14 conference, 2014.
GROS, B.; KINSHUK; MAINA, M. Preface. In: GROS, B.; KINSHUK, M. (Org.), The future of ubiquitous learning. Learning design for emerging pedagogies, New York: Springer, 2016. DOI: https://doi.org/10.1007/978-3-662-47724-3
HEGEDUS, S. J.; MORENO-ARMELLA, L. Introduction: the trans-formative nature of ‘dynamic’ educational technology. ZDM - The International Journal on Mathematics Education, v. 41, n. 4, p. 397–398, 2009. ISSN 18639704. Disponível em: https://link.springer.com/article/10.1007/s11858-009-0201-9. Acesso em: 03 jun. 2020. Doi: https://doi.org/10.1007/s11858-009-0201-9 DOI: https://doi.org/10.1007/s11858-009-0201-9
HEGEDUS, S. J.; TALL, D. O. Foundations for the future: The potential of multimodal technologies for learning mathematics. In: ENGLISH, L.; KIRSHNER, D. (Org.), Handbook of international research in mathematics education, 3rd ed., New York: Taylor & Francis, 2016.
HÖLZL, R. Using Dynamic Geometry Software to Add Contrast to Geometric Situations – A Case Study. International Journal of Computers for Mathematical Learning, v. 6, n. 1, p.63–86, 2001. ISSN 15731766. Disponível em: https://link.springer.com/article/10.1023/A:1011464425023. Acesso em: 15 jul. 2020. Doi: http://dx.doi.org/10.1023/A:1011464425023. DOI: https://doi.org/10.1023/A:1011464425023
LESH, R.; ZAWOJEWSKI, J. S. Problem solving and modelling. In: LESTER, F. (Ed.), The second handbook of research on mathematics teaching and learning, NC: Information Age Publishing, 2007.
LEUNG, A.; BOLITE-FRANT, J. Designing mathematics tasks: The role of tools. In: WATSON, A.; OHTANI, M. (Org.), Task design in mathematics education, New ICMI Study Series. New York: Springer, 2015. DOI: https://doi.org/10.1007/978-3-319-09629-2_6
LEUNG, A.; BACCAGLINI-FRANK, A. Introduction. In: LEUNG, A.; BACCAGLINI-FRANK, A. (Org.). Digital technologies in designing mathematic education tasks, Switzerland: Springer, 2017. DOI: https://doi.org/10.1007/978-3-319-43423-0
MASON, J.; JOHNSTON-WILDER, S. Designing and using mathematical tasks. St. Albans: Tarquin Publications, 2006.
NATIONAL COUNCIL OF TEACHERS OF MATHEMATICS. Principles and standards for school mathematics. Reston, VA: National Council of Teachers of Mathematics, 2000.
NATIONAL COUNCIL OF TEACHERS OF MATHEMATICS. Focus in High School Mathematics: Reasoning and sense making. Reston, VA: National Council of Teachers of Mathematics. 2009.
PÓLYA, G. How to Solve it. Princeton: Princeton University Press. 1945.
PINHO, J. L. R.; MORETTI, M. T. Estimulando a criatividade em matemática em sala de aula através da formulação e resolução de problemas em geometria. REMATEC, v. 13, n. 28, p. 55-67, 2018. ISSN 1980-3141. Disponível em: http://www.rematec.net.br/index.php/rematec/article/view/135. Acesso em: 19 jun. 2020.
POVEDA, W. Resolución de problemas matemáticos en GeoGebra. Revista do Instituto GeoGebra Internacional de São Paulo, v. 9, n. 1, p. 26-42, 2020. ISSN 2237-9657. Disponível em: https://revistas.pucsp.br/index.php/IGISP/article/view/46907. Acesso em: 18 jul. 2020. DOI: https://doi.org/10.23925/2237-9657.2020.v9i1p26-42. DOI: https://doi.org/10.23925/2237-9657.2020.v9i1p26-42
POVEDA, W.; GOMEZ-ARCIGA, A. MOOC Resolución de problemas matemáticos y uso de tecnologías digitales: Su diseño e implementación. In: ALVARADO, A.; CARMONA, G.; MATA, A. (Org.) Una visión integradora. Tópicos Selectos de Educación en CITeM, México: Ecorfan, 2017. Disponível em: https://www.ecorfan.org/actas/Una_vision_integradora/Una_visi%C3%B3n_integradora.pdf. Acesso em: 05 jun. 2020.
SANTOS-TRIGO, M.; CAMACHO-MACHÍN, M. Framing the use of computational technology in problem solving approaches. The Mathematics Enthusiast Journal, v. 10, n.1-2, p. 279–302, 2013. ISSN 1551-3440. Disponível em: https://scholarworks.umt.edu/tme/vol10/iss1/. Acesso em: 06 jun. 2020. DOI: https://doi.org/10.54870/1551-3440.1268
SANTOS-TRIGO, M.; MORENO-ARMELLA, L.; CAMACHO-MACHÍN, M. Problem solving and the use of digital technologies within the Mathematical Working Space framework. ZDM: The International Journal on Mathematics Education, v. 48, n. 6, p. 827–842, 2016. ISSN 18639704 Disponível em: https://link.springer.com/article/10.1007/s11858-016-0757-0. Acesso em: 10 jun. 2020. DOI: https://doi.org/10.1007/s11858-016-0757-0. DOI: https://doi.org/10.1007/s11858-016-0757-0
SCHOENFELD, A. Mathematical problem Solving. New York: Academic Press. 1985.
SCHOENFELD, A. Method. In: LESTER, F. (Org.), Second Handbook of Research on Mathematics Teaching and Learning, New York: MacMillan, 2007.
Descargas
Publicado
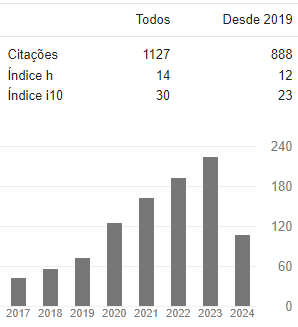
- Visualizações do Artigo 284
- PDF (Português (Brasil)) downloads: 137






 Português (Brasil)
Português (Brasil)
 Español (España)
Español (España)
 English
English